A Möbius strip is nothing more than a loop with a half-twist…
…but it is really weird! Make one and see for yourself.
It only has one edge and one side.
What happens if you cut it along the middle?
Do you get two loops?
No, just one. But it is not a Möbius band itself.
And if you cut it again?
This time you get two entangled loops.
www.sciensation.org | Ciênsação hands-on experiments are published as Open Educational resources under a Creative Commons Attribution-ShareAlike 4.0 International License.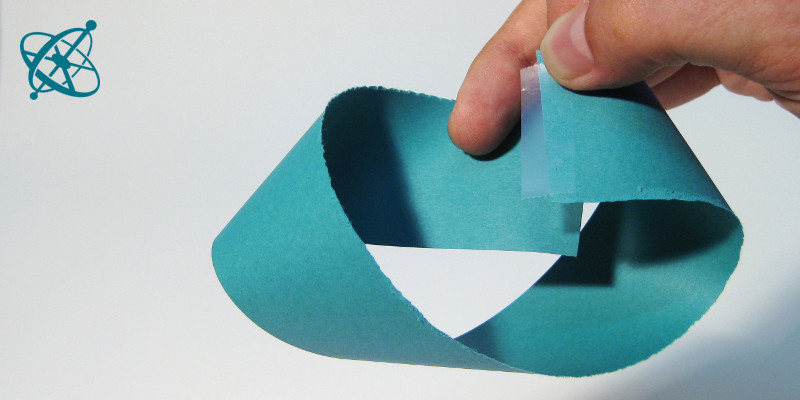
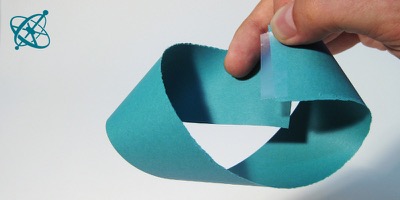
A Möbius strip is nothing more than a loop with a half-twist…
…but it is really weird! Make one and see for yourself.
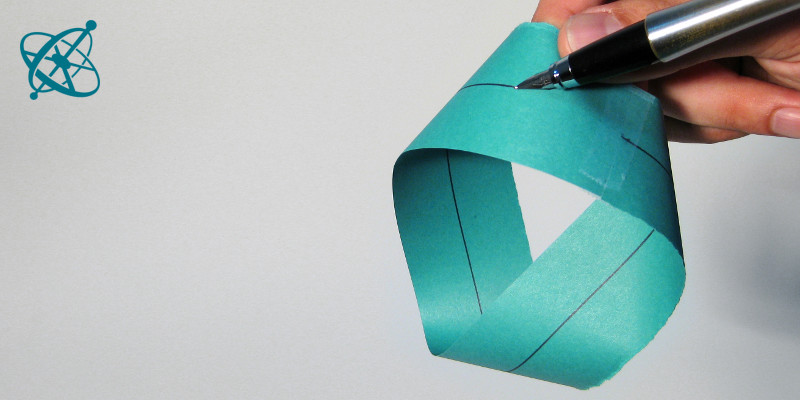
It only has one edge and one side.
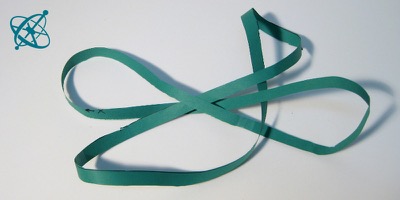
What happens if you cut it along the middle?
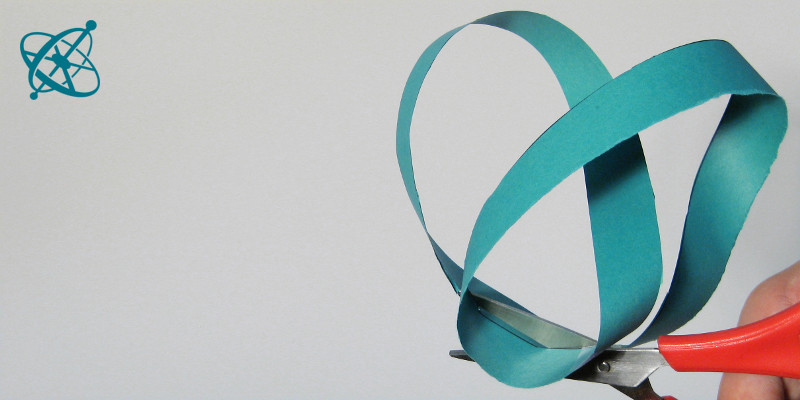
Do you get two loops?
No, just one. But it is not a Möbius band itself.
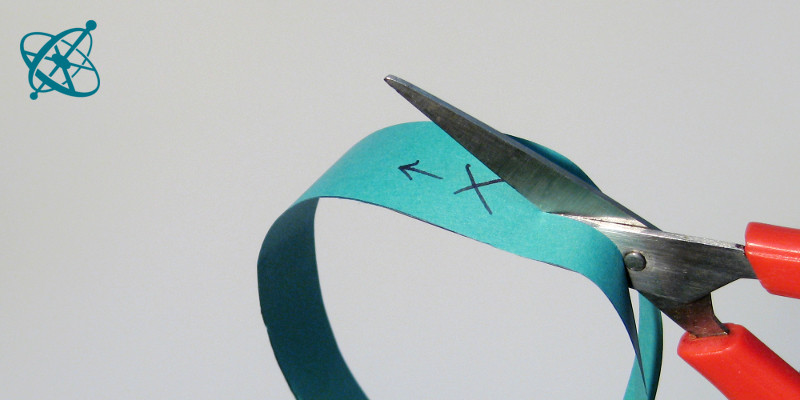
And if you cut it again?
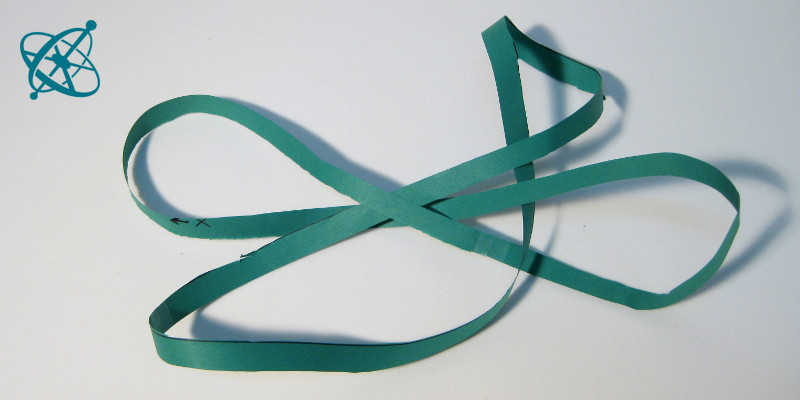
This time you get two entangled loops.
Not everything has two sides
The Möbius strip and its unusual properties are puzzling if not irritating. It is a very good example that illustrates how fast we leave familiar ground and confront difficulties to truly comprehend what's going on. As simple as its form may seem, as hard it can be to predict the outcome of even basic activities – as e.g. cutting it along its middle.
A Möbius strip is a three-dimensional object with only one side and one edge.
Math is full of surprises.
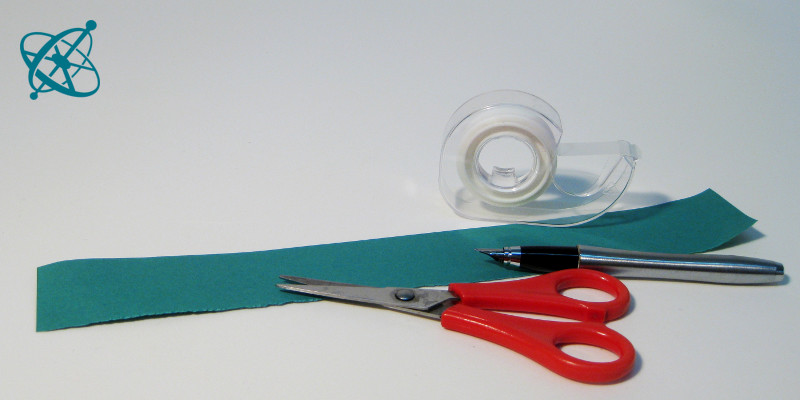
Sticky tape
Scissors
Pencil
Cut an about 4 cm wide stripe of paper from the long side of an A4 sheet and twist one end before taping the ends together to a loop, or, more specifically, a Möbius band.
1. How many sides and edges has this band? How do you proof this?
2. How many loops do you get if you cut the band along the middle of the stripe? Try it.
3. How many loops do you get if you cut the band again along the middle of the stripe? Try it.
4. What makes this band so strange?
If you draw a line along the the middle of the strip until you come back from where you started, what do you note?
› The marking is on 'all' sides, meaning the Möbius strip has only one.
If you mark one edge along the band until you come back to where you started, what do you note?
› 'All' edges are marked, meaning the Möbius strip has only one.
A Möbius strip has only one side and only one edge. By cutting it along the middle of the stripe, a second edge is added and the result is a single loop that has no longer the properties of a Möbius strip. Repeating the cutting along the middle leads to two interlocked loops.