 www.sciensation.org | Ciênsação hands-on experiments are published as Open Educational resources under a Creative Commons Attribution-ShareAlike 4.0 International License.
www.sciensation.org | Ciênsação hands-on experiments are published as Open Educational resources under a Creative Commons Attribution-ShareAlike 4.0 International License.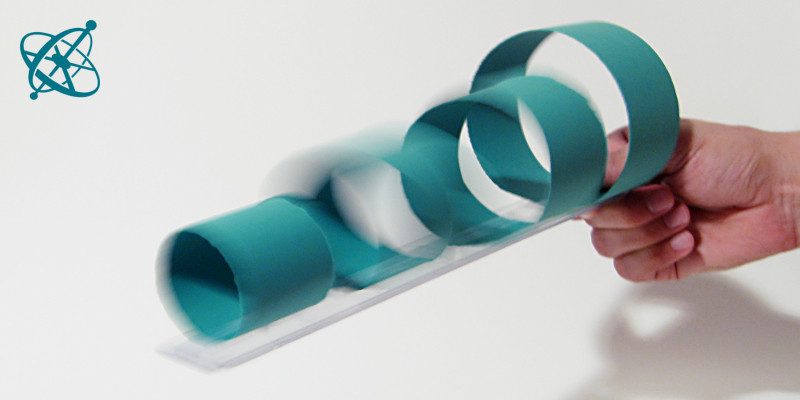
Can you find the resonance frequency?
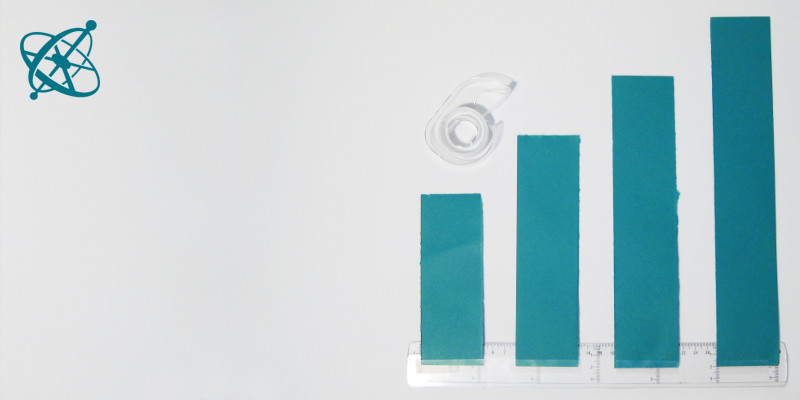
Tape stripes of different length to a ruler,…
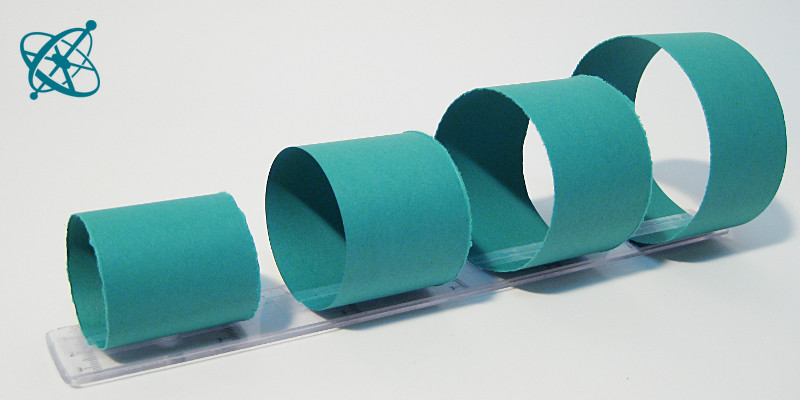
…and fix them as loops.
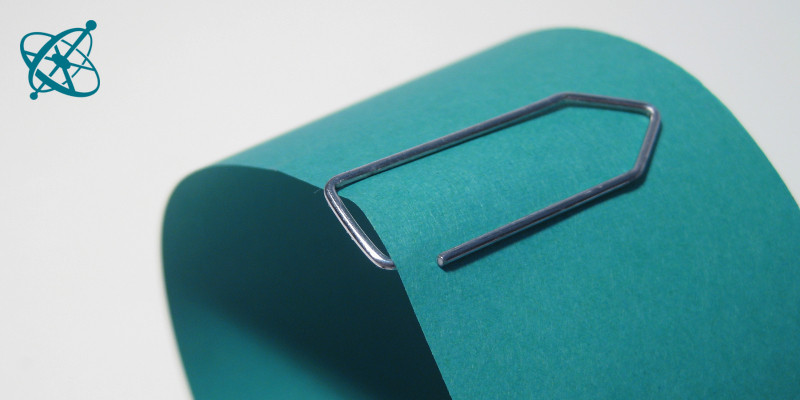
What changes with additional mass?

An effect that is also used to stabilize buildings against Earth quakes.
Wobbling loops
Mechanical systems, be it a tiny clockwork or a skyscraper, have a resonance frequency that depends on stiffness, mass and other parameters. Paper loops of different sizes build a simple and safe model to get a feeling for this ubiquitous phenomena.
Finding the resonance frequency of a mechanical system.
Observing how mass can be used to damp oscillations.
Sheet of paper
Sticky tape
Paper clips
Make four paper loops of different sizes and attach them to a ruler. Then slowly shake the ruler sideways, constantly increasing the frequency of your shaking.
1. When does each loop vibrate particularly strongly?
2. If you attach a paperclip to a loop, how does the swinging behavior change?
If you press slightly on the top of each loop, what difference do you note?
› Larger loops are easier deformed than smaller ones, meaning they have a smaller stiffness.
What are the differences between the loops?
› The loops differ in size, mass and stiffness. The larger the diameter, the higher the mass and the lower the stiffness.
The largest loop will begin to swing strongly at a low frequency. As you increase the frequency, the largest loop moves less and the next smaller one begins to vibrate – each according to its own resonance frequency which depends on the respective mass and stiffness.
Attaching a paper clip not only increases the total mass – and thereby lowers the resonance frequency – but also adds inertia at the top, changing the oscillation modes of the loop. You can see this clearly when attaching the paper clip to another part of the loop. This effect is used to dampen oscillations, e.g. to stabilize skyscrapers when the ground is shaking: In the Taipai 101 (Taiwan, height 508m) a tuned mass damper (660 tons) near the top sways like a pendulum to offset movements in the building.




