How is the angle…
…between two mirrors related…
…to the number of copies…
…you see of a single object?
www.sciensation.org | Ciênsação hands-on experiments are published as Open Educational resources under a Creative Commons Attribution-ShareAlike 4.0 International License.
How is the angle…
…between two mirrors related…
…to the number of copies…
…you see of a single object?
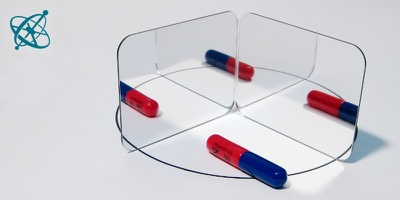
Mirror fractions
Two mirrors at an acute angle have an almost magical effect on your students' attention. By simply drawing a circle under the mirrors, you can create an opportunity for your students to discover the principles of multiple reflections and how they relate to fractions.
Observing and describing regularities in a phenomena.
Understanding the effects of multiple reflections.
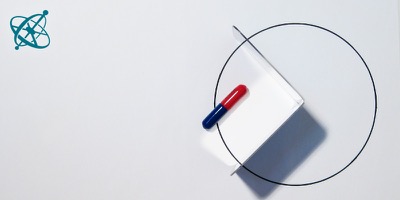
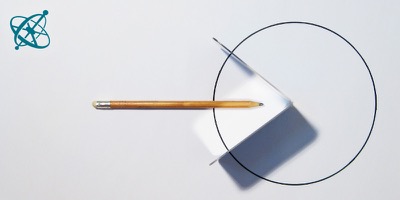
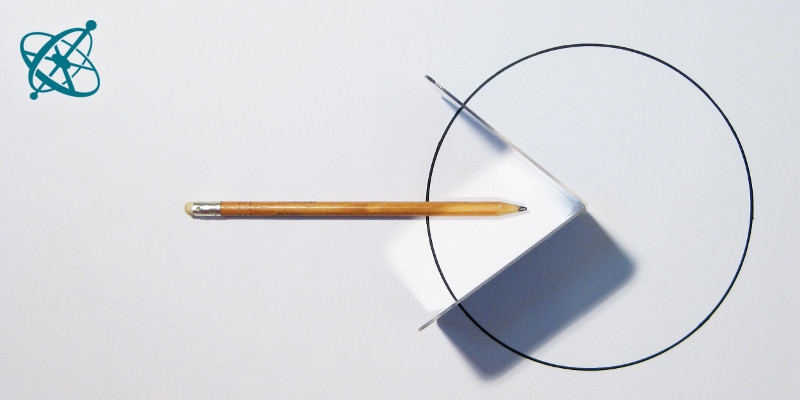
Choose a round object, e.g. a dish, with a radius a bit smaller than the side length of your mirrors. Let students use this object to draw circles on a sheet of paper.
Connecting two mirrors with a bit of sticky tape on their back simplifies their handling.
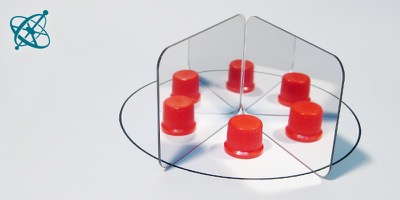
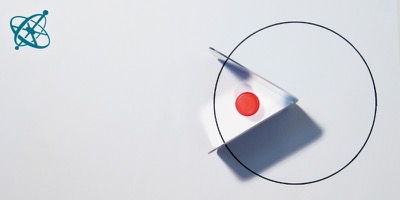
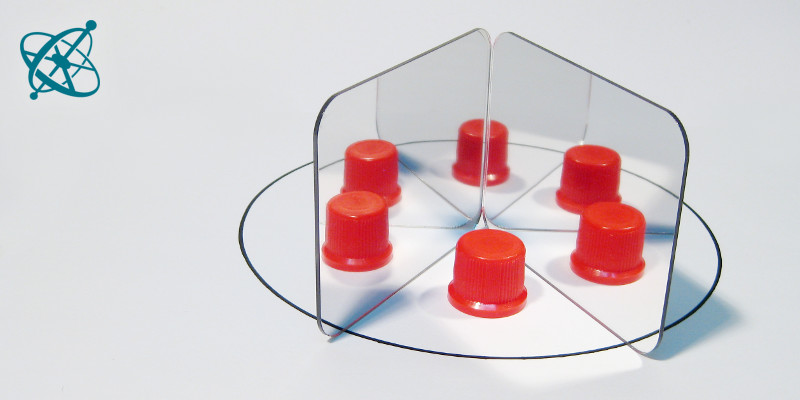
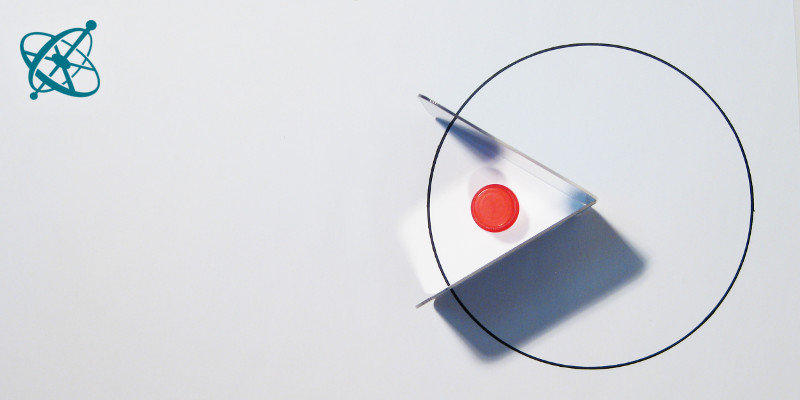
Hold two mirrors so that their edges touch at the center of the circle and place a small object between them.
1. What do you note about the circle you see in the mirrors?
2. Can you find the rule that determines how many images of the object you get to see in the mirrors?
Your students will quickly note that the circle always seems complete, even though only a part of it lies between the mirrors.
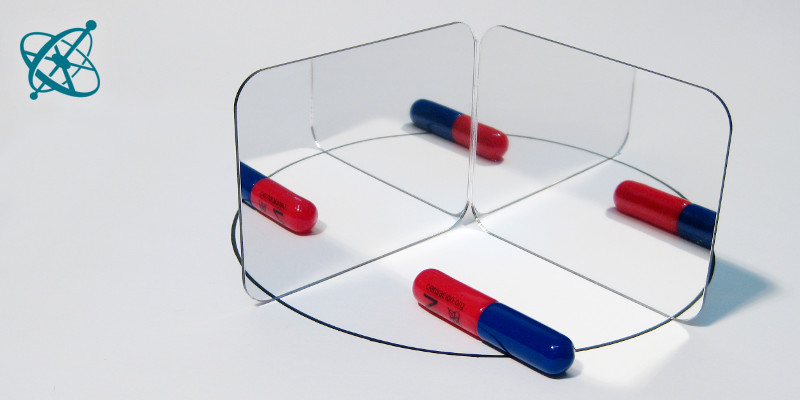
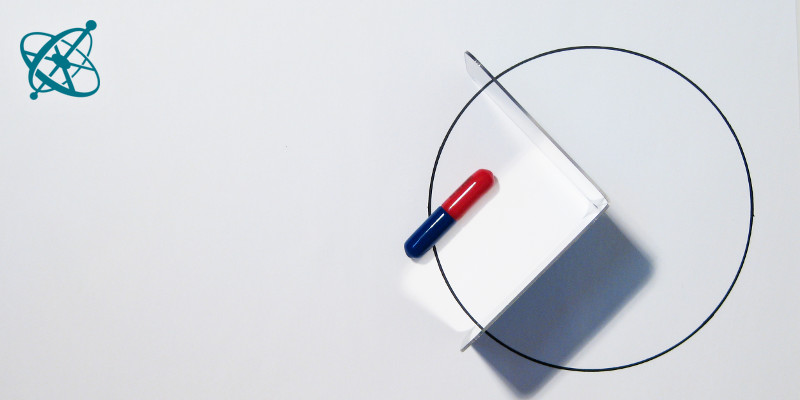
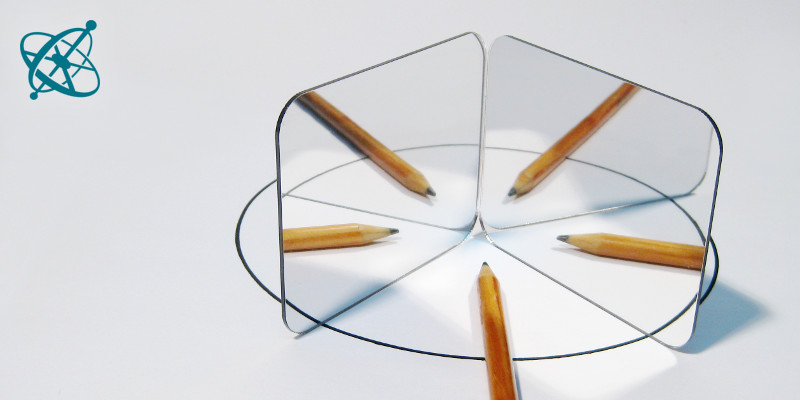
The number of images they see corresponds to the fraction of the circle the two mirrors encompass. If the area between the mirrors is less than 1/2 and more than 1/3, they can see 2 images of the object; between 1/3 and a 1/4 of the area, there are 3 images, and so on.